One Student of 10th Standard / 10th grade asked me one question. I would like to share the method of solving the problem & its presentation with you. Please go through it & make the habit of applying the same technique to solve other problems & please share this technique with your friends & relatives. (Please note one thing that “By distributing our knowledge, it will boost & by keeping it only with us, it will reduce”).
The Problem is very simple. Please see the following Problem.
The Shape of the wooden Block is of the form of a frustum of a cone. Its Volume is 56980 cubic centimeters. If the radii of the top and bottom are 28 cm and 21 cm respectively, then find its height. (π = 22/7) (Note: Don’t use the calculator) Please see the following diagram.
Actually, this problem is very simple. But I would like to tell you the simple method of calculations & the proper method of presentation of this problem on paper.
First I would like to discuss the presentation, which will be useful for all the problems.
I would like to give numbers to each step. These numbers are to be written to the left of each step. Secondly, the equation numbers are to be given only the equations & to be written to the right of the equations. You can see the above problem which is solved by using the same technique.
Now we will discuss the basics of the problem.
Here we know that the volume of the frustum is
Here we want to find height so
So many students will solve this problem by taking the product of numerator & the product of Denominator & then take the division. I had done this problem with the different method of calculations which is very simple, saves your valuable time and authentic also.
First I will show how some student solves this problem & then I will explain its simple method of calculations so that you will definitely find the difference. I am sure that you will like this method in which you never require to do much more complicated calculations.
(Note: The description written in the box bracket in BLACK is only to understand the steps & not to be written in the solution of the problem)
So many Students may solve this problem by the following method
Note: You may observe fewer steps in this method but you will spend more time of Multiplication, Squaring the numbers 28 & 21 then the time of addition. This thing should be noted in my method given below.
Note: You may observe fewer steps in this method but you will spend more time of Multiplication, Squaring the numbers 28 & 21 then the time of addition. This thing should be noted in my method given below.
Given: 1) V = 56980
2) Radius of Top (Larger Circle
3) Radius of Bottom (Smaller Circle
4) π = 22/7
Find: Height h
1) We know that,
[Here Students must take the complete square of 28 and 21 and the product of 28 and 21 as shown below
[Multiplying Numerator & denominator by 7 write down the addition of all three terms of the square bracket]
[Then the student will divide numerator and denominator by 7 and write the results]
[Divide Numerator & Denominator by 37 and write the results]
h = 3 x 10
So Height of the Wooden Block is h = 30 cm.
Please see the following simple method of calculations. It will definitely save your time & you will definitely like this method
Given: 1) V = 56980
2) Radius of Top (Larger Circle
3) Radius of Bottom (Smaller Circle
4) π = 22/7
Find: Height h
1) We know that,
[Here modify the term of the denomination using the formula [r12 + r22 – 2 x r1 x r2 = (r1 - r2) 2. so we can write [r12 + r22 + (r1 x r2)] = r12 + r22 – 2 x r1 x r2 + 2x r1 x r2 + r1 x r2] so we have]
[Here modify the term of the denomination using the formula [r12 + r22 – 2 x r1 x r2 = (r1 - r2) 2. so we can write [r12 + r22 + (r1 x r2)] = r12 + r22 – 2 x r1 x r2 + 2x r1 x r2 + r1 x r2] so we have]
[Take 7 common from the denominator, we get]
[Take 7 common from the denominator, we get]
[Again divide Numerator & Denominator by 7, we get]
So Height of the Wooden Block is h = 30 cm.
Like this, you can also find so many ways of simple calculation methods and apply the same to other problems. At present, you may feel that these calculations are difficult. But while doing more practice of applying this technique, you may be more comfortable to use these simple calculation methods.
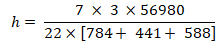
No comments:
Post a Comment